Option delta explained
for example, Stock option price If the underlying stock price rises by 1 cent, the option’s delta will be 0.5 if the price rises by 0.5 cents.
Another way to look at delta is as the probability that a money option will expire.
Delta neutral strategies include ATM long straddles, long strangles and calendar spreads.
Option delta operation
You don’t need to understand the math behind delta (go on to the next section if you want), but for those interested, delta is more formally the partial derivative of the option price relative to the underlying price. Defined.
The formula is (understanding the normal distribution requires some knowledge of normal distributions):
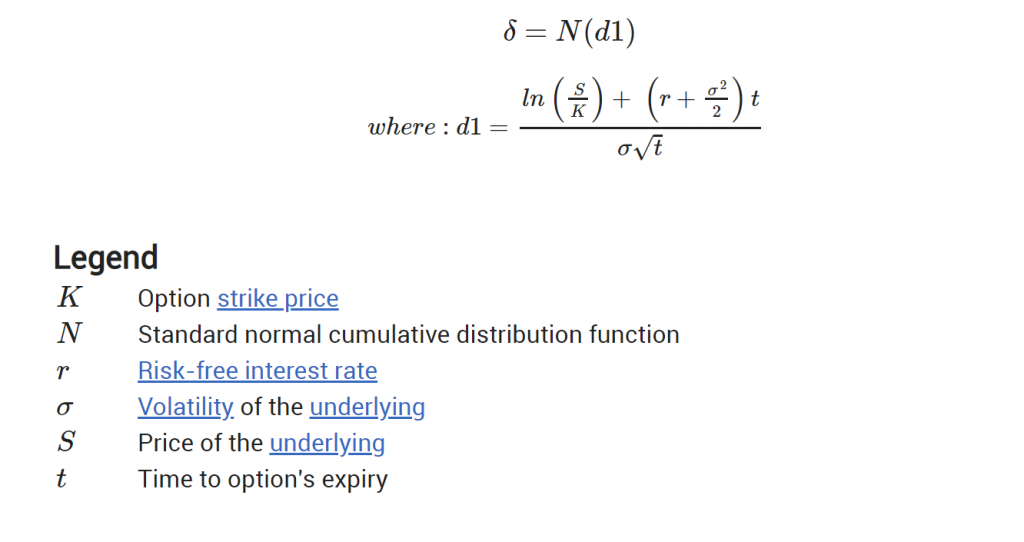
sauce: iota finance
Delta is ostensibly the most intuitive of the Greek options. Even the newest novice would expect the price of an option that gives the right to buy or sell a particular security to change with the price of the security.
Let’s take an example where you have a call option on a stock with a stock price of $120 and as the stock price increases (say from $10 to $130).
In-the-money options – strike price For less than $120, you save even more. Therefore, their value to the holder increases and the probability that they remain in the money increases, thus increasing the price of the option, all other things being equal.
Out-of-the-money options and at-the-money options, i.e. options with a strike price of $120 or more, will also appreciate. For example, the probability of a $140 option expiring in-the-money is higher if the stock is $130 than if the stock is $120. Therefore its value is higher.
Similar arguments can be used with put options. Its value rises/falls according to the fall/rise of the underlying (the only difference is that the delta for put options is negative, while the delta for call options is positive).
But the range of this sensitivity, or delta, and how it relates to expiry length, price, and volatility is very nuanced. Let’s take a closer look.
delta for short and long options
Options can be bought or sold. Depending on which side of the option trade the investor is on, the delta for that option is adjusted accordingly.
For long options, the delta value is positive for calls and negative for puts. Purchased (long) calls have a delta between 0 and +1, which increases as the option’s in-the-money increases. Purchased put options have a delta between 0 and -1, with the delta decreasing the more in-the-money the put is.
The opposite is true when shorting options. If you sell a call option, the delta score will be a negative value between 0 and -1. This is true, a short call option position increases in value as the underlying security falls. Call option creators can profit as the underlying security falls. Another way to capture this is to understand that a call option has a positive delta, but the seller/seller of that call option has reverse exposure.
Similarly, a put option that offers the holder a delta exposure of -1 to 0 will give the put option seller/writer a positive delta of between 0 and +1.

How does option delta change over time?
The impact of time on delta depends on the “financial value” of the option.
in the money
All other things being equal, long-term in-the-money options have a lower delta than short-term ones.
In-the-money options have both intrinsic value (share price minus strike price) and extrinsic value.
Over time, the extrinsic value decreases (due to theta) and the intrinsic value (which moves with the stock price) becomes more dominant. Therefore, its delta increases towards 1 over time as the option moves more in line with the stock price.
I’m out of money
All other things being equal, the delta for the short dated OTM/ATM option is lower than the long dated one.
Short-term out-of-the-money options (especially those that are significantly OTM) are unlikely to expire in-the-money, and this fact can change with every penny change in price. is low. So its delta is low.
Long-dated OTM options are more likely to expire in-the-money, increasing the time it takes for options to migrate to ITM. Therefore, its value fluctuates according to the stock price. So their delta will be higher.
with money
At-the-money option deltas are not time sensitive.
How does option delta change with implied volatility?
Again the effect implied volatility Delta change depends on monetary value.
in the money
As we saw above, the value of a money option consists of both intrinsic and extrinsic amounts.
In general, the higher the ratio of an option’s intrinsic value (which fluctuates exactly with the stock price) to its extrinsic value (which does not move with the stock price), the higher its delta.
Since increasing the IV increases the option’s extrinsic value, the intrinsic value is not affected by the implied volatility, thus increasing the option’s extrinsic value percentage. The resulting reduction in intrinsic value of the total reduces the option’s delta as shown above.
out of the money
Out-of-the-money options have only external value, which is determined by the probability of expiring in-the-money.
Higher volatility suggests that options are more likely to expire ITMs (because stocks are expected to move more volatile), thus increasing delta.
with money
ATM options have a delta of about 100 minutes. 0.5, does not change as volatility changes.
Impact of price changes on Delta Air Lines
Another subtlety of deltas is that the value of the delta itself changes as the price of the underlying security changes.
The extent to which this occurs is gamma, one of the Greek options. This is the delta change resulting in a 1c change in the stock price.
Long option holders have a positive gamma, while short positions have a negative gamma. That is, it helps the former and penalizes the latter. Also, the closer the deadline is, the higher the absolute value. (See here for more information on gamma).
Conclusion
Delta is an important Greek word because it reflects the option holder’s exposure to one of the key variables, the price of the underlying security.
It is one of the easiest option concepts to understand, but the behavior resulting from changes in other variables such as time, IV, base price, etc. is more complex.
Understanding these concepts is very important for options traders.
About the Author: Chris Young has a degree in Mathematics and 18 years of experience in finance. Chris is British, but he has worked in the US and most recently in Australia. His interest in options was first sparked by the “Trading Options” section of the Financial Times of London. He was determined to pass this knowledge on to a wider audience and in 2012 founded Epsilon He Option.
Related article:

